Vector and Geometric Calculus
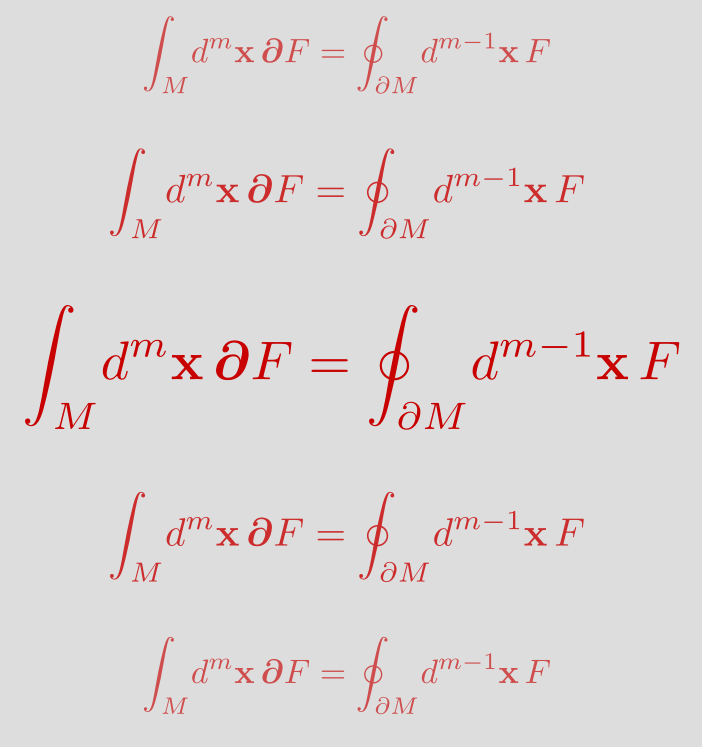
Contents
- Preliminaries
- Curve and Surface Representations
- Limits and Continuity
- Derivatives
- The Differential
- Tangent Spaces
- The Gradient
- Extrema
- Integrals
- Integrals over Curves
- Multiple Integrals
- Integrals over Surfaces
- The Fundamental Theorem of Calculus
- The Fundamental Theorem of Geometric Calculus
- Differential Geometry
- Differential Geometry in ℝ3
- Appendices
Read a detailed table of contents, the preface, and the index.
This textbook for the undergraduate vector calculus course presents a unified treatment of vector and geometric calculus. It is a sequel to my Linear and Geometric Algebra. That text is a prerequisite for this one.
Linear algebra and vector calculus have provided the basic vocabulary of mathematics in dimensions greater than one for the past one hundred years. Just as geometric algebra generalizes linear algebra in powerful ways, geometric calculus generalizes vector calculus in powerful ways.
Traditional vector calculus topics are covered, as they must be, since readers will encounter them in other texts and out in the world.
The book can be used for self study by those comfortable with the theorem/proof style of a mathematics text.
Suggestions for instructors (one page).
Current Printing
The latest printing is from April 2025.
There are minor improvements and corrections.
Videos
I have created a five video YouTube playlist Geometric Calculus, about 53 minutes in all, taken from the book. It is a sequel to my Geometric Algebra playlist. Some knowledge of vector calculus is a prerequisite for the videos, but no knowledge of geometric calculus is assumed. The book assumes no knowledge of vector calculus.
What People Are Saying
From a review of Linear and Geometric Algebra:
"Alan Macdonald's text is an excellent resource if you are just beginning the study of geometric algebra and would like to learn or review traditional linear algebra in the process. The clarity and evenness of the writing, as well as the originality of presentation that is evident throughout this text, suggest that the author has been successful as a mathematics teacher in the undergraduate classroom. This carefully crafted text is ideal for anyone learning geometric algebra in relative isolation, which I suspect will be the case for many readers."
— Jeffrey Dunham, William R. Kenan Jr. Professor of Natural Sciences, Middlebury College
Available at Amazon
Vector and Geometric Calculus is available at Amazon. See the strong reviews there.
Price: $39
Computer Exercises
GAlgebra. The computer exercises in the book use GAlgebra,
a Python 3 module for symbolic geometric algebra and calculus calculations.
It is cross-platform (Linux, PC, Mac), with all components freely available on the web.
GAlgebra runs in a browser using Jupyter Lab notebooks.
Output is typeset in beautiful LaTeX.
See Linear and Geometric Algebra's web page for instructions for obtaining and using GAlgebra.
Contact
Please email me corrections, typos, or any other comments about the book. If appropriate, I will change the book in the next printing.