The figure above shows the temperature contours in black solid lines. The dashed lines represent a uniform grid with a spacing of 100 km in both the $x$ and $y$ directions. The temperature gradient is a vector whose magnitude is the rate of change of temperature with distance $\frac{\Delta T}{\Delta d}$ and points in the direction of greatest increase in temperature. Because temperature would not change if one were to move along the temperature contour, (That is, move in a direction tangent to the contour at that point), the direction of greatest increase is perpendicular to the temperature contour at the location where the gradient is determined.
The magnitude of the temperature gradient at station 1 is
$\frac{\Delta T}{\Delta d} = \frac{40 - 30}{200} = 10/200 = 0.05$ F/km = $0.00005 = 5.0 \times 10^{-5}$ F/m
The magnitude of the temperature gradient at station 2 is
$\frac{\Delta T}{\Delta d} = \frac{55 - 45}{400} = 10/400 = 0.025$ F/km = $0.000025 = 2.5 \times 10^{-5}$ F/m.
Temperature gradients and wind vectors can combine to create temperature advection resulting in a time-rate of change of temperature at a given location. The rate of change of temperature with respect to time at a given location may be estimated using the temperature gradient and the wind vector. The formula is
$\frac{\Delta T}{\Delta t} = -\frac{\Delta T}{\Delta d} \cdot \frac{\Delta d}{\Delta t} \cdot \cos \theta = -\frac{\Delta T}{\Delta d} \cdot s \cdot \cos \theta$,
where $\theta$ is the angle between the temperature gradient vector and the velocity vector, and $s$ is the speed (magnitude) of the wind given by $s = |\vec{v}| = \sqrt{u^2 + v^2}$. Letters $u$ and $v$ represent the wind components in the $x$ direction and $y$ directions, respectively. Note that the temperature gradient must have units of degrees per meter.
The minus sign is required to make the temperature tendency correct. If the wind is blowing parallel to, and in the direction of the temperature gradient, then the temperature at a location downwind will decrease with time because cooler air is replacing warmer air.
As an example, suppose the wind at station 1 is westerly with a speed of 10 m/sec. Then $\vec{v} = (10,0)$ and $s = \sqrt{10^2 + 0^2} = 10$. The velocity vector aligns with the temperature gradient, so the angle between them is $\theta = 0$. Therefore,
$\frac{\Delta T}{\Delta t} = -\frac{1}{1000} 0.05 \cdot 10 \cdot \cos 0 = -0.00005$ F/sec. = $-0.00005 \cdot 60 \cdot 60$ = -1.8 F/hour
The factor of $\frac{1}{1000}$ converts the temperature gradient to degrees/meter. The two factors of "60" are used to convert the rate of change from F/sec to F/hour because there are 60 seconds per minute and 60 minutes per hour.
As another example, suppose the wind at station 1 is southerly with a speed of 10 m/sec. Then $\vec{v} = (0,10)$ and $s = \sqrt{0^2 + 10^2} = 10$. The velocity vector is perpendicular with the temperature gradient, so the angle between them is $\theta = 90$. Therefore, $\cos \theta = \cos 90 = 0$, and
$\frac{\Delta T}{\Delta t} =-\frac{1}{1000} 0.05 \cdot 10 \cdot 0 $= 0 F/hour
As another example, suppose the wind at station 1 is southwesterly, with $\vec{v} = (7,7)$. Here, and $s = \sqrt{7^2 + 7^2} = \sqrt{2} \cdot 7 \approx 9.9$ m/sec. The velocity vector and the temperature gradient vector form an angle of 45 degrees, so $\cos \theta = \cos 45 = \sqrt{2}/2$.
$\frac{\Delta T}{\Delta t} =-\frac{1}{1000} 0.05 \cdot \sqrt{2} \cdot 7 \cdot \sqrt{2}/2 \cdot 60 \cdot 60 $= -1.26 F/hour
As another example, suppose the wind at station 1 is easterly with a speed of 10 m/sec. Then $\vec{v} = (-10,0)$ and $s = \sqrt{(-10)^2 + 0^2} = 10$. The velocity vector is parallel with the temperature gradient, but in the opposite direction, so the angle between them is $\theta = 180$. Therefore, $\cos \theta = \cos 180= -1$, and
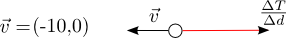
$\frac{\Delta T}{\Delta t} =-\frac{1}{1000} 0.05 \cdot 10 \cdot \cos 180 = 0.00005$ F/sec. = $0.00005 \cdot 60 \cdot 60$ = 1.8 F/hour