Pressure Gradient

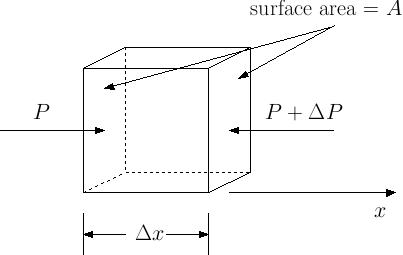
The figure below shows an arbitrary air parcel located on an arbitrary surface.

symbol definition units

$A$ vertical cross-section of the parcel $L^2$

$\Delta x$ length of the parcel $L$

$P$ pressure magnitude $\frac{F}{L^2}$

$\Delta P$ change in pressure magnitude $\frac{F}{L^2}$

$V$ volume of the parcel $L^3$

$m$ mass of the parcel $m$

$\rho$ density of the parcel $\frac{m}{L^3}$

$a$ acceleration of the parcel $\frac{L}{time^2}$

symbol	definition	units
$A$	vertical cross-section of the parcel	$L^2$
$\Delta x$	length of the parcel	$L$
$P$	pressure magnitude	$\frac{F}{L^2}$
$\Delta P$	change in pressure magnitude	$\frac{F}{L^2}$
$V$	volume of the parcel	$L^3$
$m$	mass of the parcel	$m$
$\rho$	density of the parcel	$\frac{m}{L^3}$
$a$	acceleration of the parcel	$\frac{L}{time^2}$

Apply Newton's second law:

$ma = \Sigma F$ $\Rightarrow$ $ma = -(P + \Delta P)\cdot A + P\cdot A $

$\Rightarrow$ $\rho V a = -P\cdot A - \Delta P\cdot A + P\cdot A $

$\Rightarrow$ $\rho A\Delta x a = - \Delta P\cdot A$

$\Rightarrow$ $a = -\displaystyle{\frac{1}{\rho}\frac{ \Delta P}{\Delta x}}$

The ratio $\frac{\Delta P}{\Delta x}$ is the pressure gradient in the $x$ direction. The minus sign indicates the direction of the acceleration is opposite the direction of increasing pressure. Note that the parcel's acceleration is inversely proportional to its density. That is, the greater the parcel's density, the lesser the acceleration. This is not too surprising... given the same pressure gradient force, a beach ball will be accelerated more than a bowling ball.

$ma = \Sigma F$	$\Rightarrow$	$ma = -(P + \Delta P)\cdot A + P\cdot A $
	$\Rightarrow$	$\rho V a = -P\cdot A - \Delta P\cdot A + P\cdot A $
	$\Rightarrow$	$\rho A\Delta x a = - \Delta P\cdot A$
	$\Rightarrow$	$a = -\displaystyle{\frac{1}{\rho}\frac{ \Delta P}{\Delta x}}$